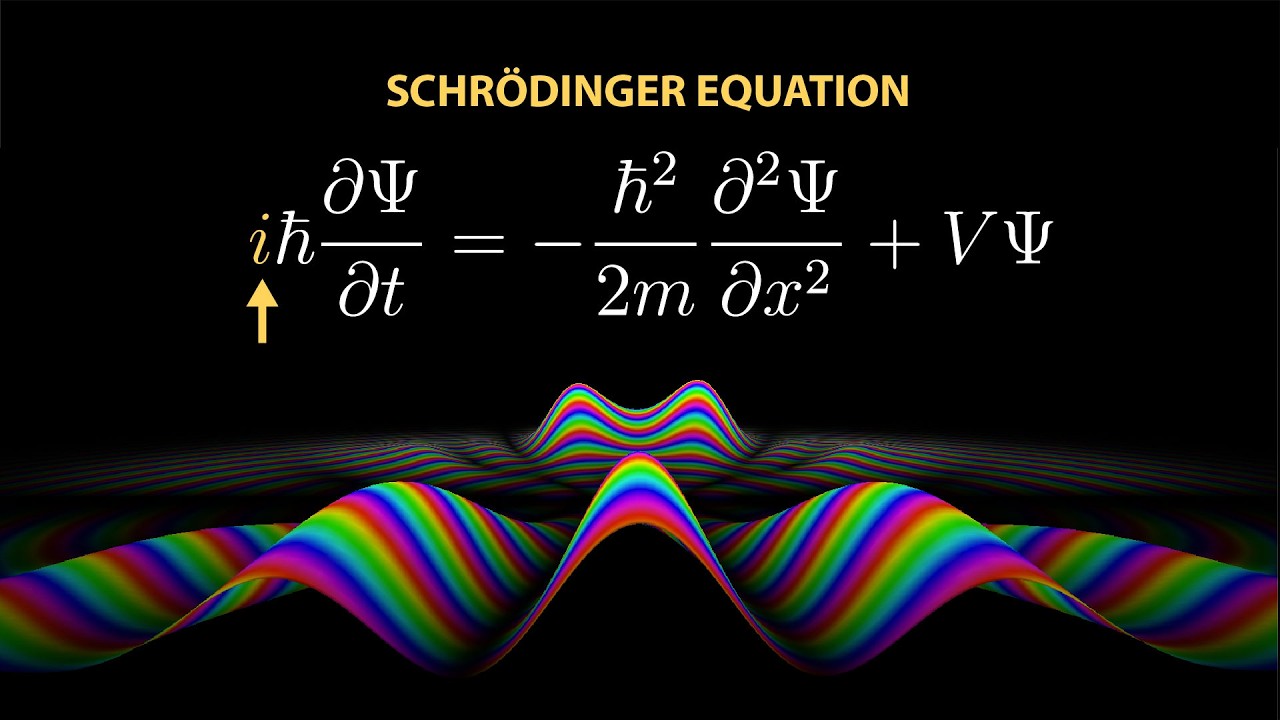
The video explains the significance of the imaginary number (i) in Schrödinger’s equation, which transformed quantum mechanics by treating particles as waves and allowing for a complex representation of quantum states. This complexity is essential for modeling interference effects and the probabilistic nature of particles, ultimately reshaping our understanding of atomic behavior and laying the groundwork for modern quantum theory.
In early 1926, Erwin Schrödinger published groundbreaking papers that transformed the understanding of physics, particularly at the atomic scale. His work introduced the Schrödinger equation, which replaced Newton’s Second Law for particles like electrons, providing an accurate description of their behavior. The equation is reminiscent of classical wave and heat equations but incorporates the imaginary number (i), raising questions about its role in quantum mechanics. Schrödinger’s equation fundamentally shifts the perspective from viewing particles as discrete entities to understanding them as waves, suggesting that the value of a matter wave at a given point in space changes over time in relation to the wave’s curvature.
The introduction of imaginary numbers in Schrödinger’s equation is significant, as it allows for a more nuanced representation of quantum phenomena. The equation’s structure implies that the wave function, which describes the state of a quantum system, must be complex rather than purely real. This complexity is essential for accurately modeling the behavior of particles, as it enables the description of interference effects and the probabilistic nature of quantum mechanics. The use of complex exponentials simplifies the mathematics involved in solving the equation, allowing physicists to derive meaningful insights about atomic behavior.
Schrödinger’s journey began with the work of Louis de Broglie, who proposed that matter could be treated as waves. Inspired by de Broglie’s ideas, Schrödinger sought to develop a wave equation for matter that would align with observed phenomena, such as the quantized energy levels of the hydrogen atom. By modifying the classical wave equation and incorporating de Broglie’s matter wave relationship, Schrödinger successfully derived an equation that matched the emission spectrum of hydrogen, demonstrating the wave-like properties of electrons.
The mathematical elegance of Schrödinger’s equation lies in its linearity, allowing for the superposition of wave functions. This property is crucial for explaining interference patterns observed in experiments like the double-slit experiment, where particles such as electrons exhibit wave-like behavior. The complex nature of the wave function captures both the amplitude and phase of the matter waves, with the square of the amplitude corresponding to the probability of finding a particle in a given location. This probabilistic interpretation, later formalized by Max Born, highlights the fundamental role of complex numbers in quantum mechanics.
In conclusion, the incorporation of imaginary numbers into Schrödinger’s equation represents a profound development in the understanding of quantum mechanics. It not only provides a mathematical framework for describing the behavior of particles at the atomic scale but also reveals the intricate relationship between waves and particles. The use of complex numbers, once deemed “imaginary,” has become essential in capturing the nuances of quantum phenomena, illustrating the unexpected ways in which mathematics can illuminate the workings of nature. Schrödinger’s work, along with the contributions of others, laid the foundation for modern quantum theory, reshaping our comprehension of the physical world.